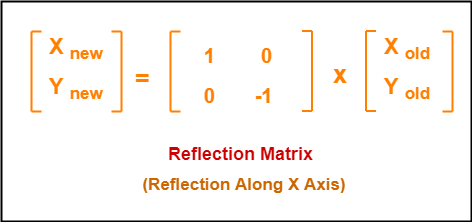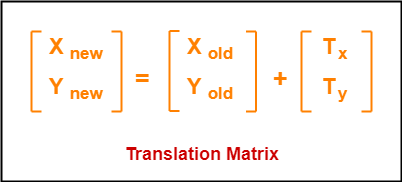2D Transformations in Computer Graphics-
We have discussed-
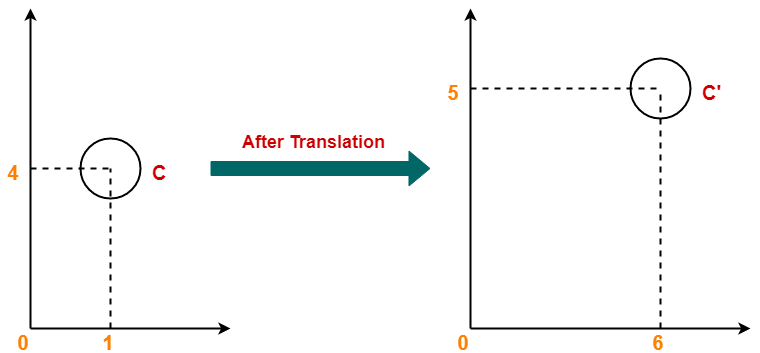
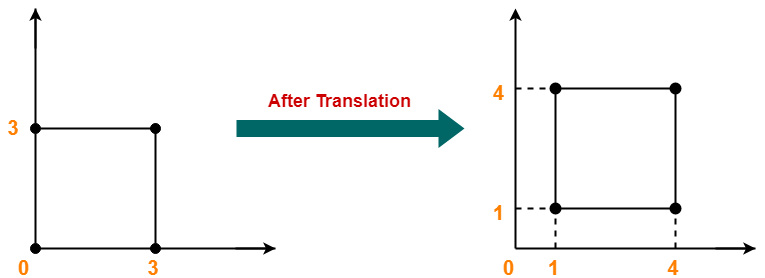
- Transformation is a process of modifying and re-positioning the existing graphics.
- 2D Transformations take place in a two dimensional plane.
In computer graphics, various transformation techniques are-
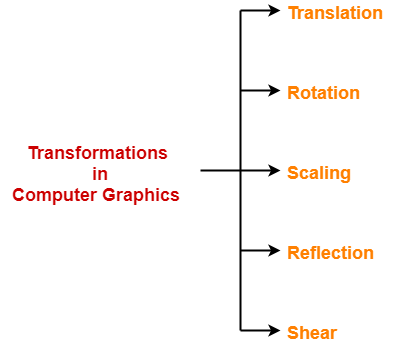
In this article, we will discuss about 2D Shearing in Computer Graphics.
2D Shearing in Computer Graphics-
| In Computer graphics,
2D Shearing is an ideal technique to change the shape of an existing object in a two dimensional plane. |
In a two dimensional plane, the object size can be changed along X direction as well as Y direction.
So, there are two versions of shearing-

- Shearing in X direction
- Shearing in Y direction
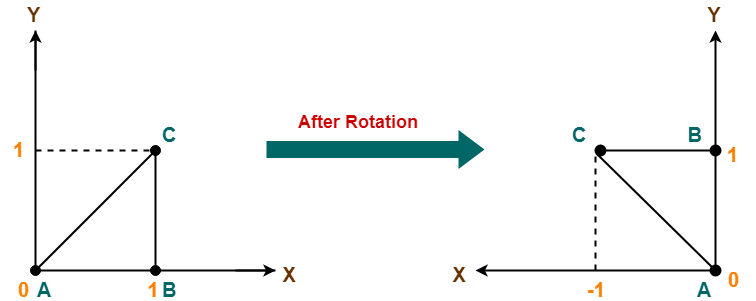
Consider a point object O has to be sheared in a 2D plane.
Let-
- Initial coordinates of the object O = (Xold, Yold)
- Shearing parameter towards X direction = Shx
- Shearing parameter towards Y direction = Shy
- New coordinates of the object O after shearing = (Xnew, Ynew)
Shearing in X Axis-
Shearing in X axis is achieved by using the following shearing equations-
- Xnew = Xold + Shx x Yold
- Ynew = Yold
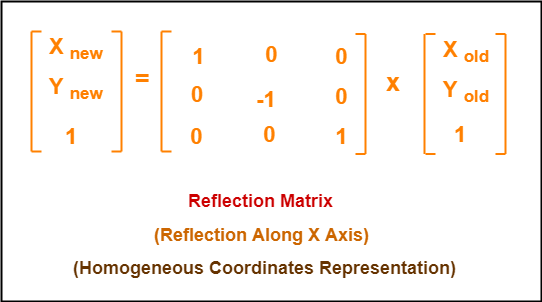
In Matrix form, the above shearing equations may be represented as-
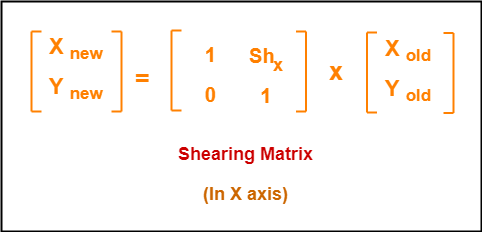
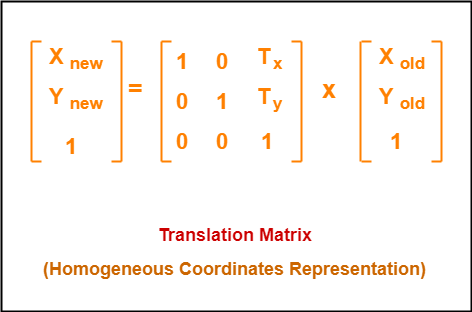
For homogeneous coordinates, the above shearing matrix may be represented as a 3 x 3 matrix as-
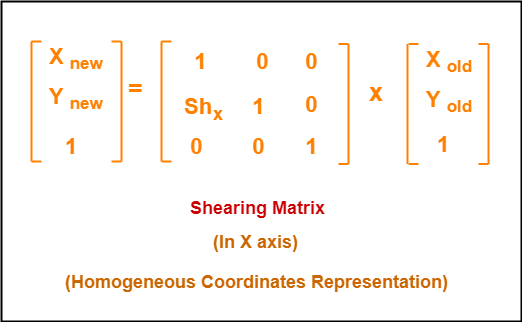
Shearing in Y Axis-
Shearing in Y axis is achieved by using the following shearing equations-
- Xnew = Xold
- Ynew = Yold + Shy x Xold
In Matrix form, the above shearing equations may be represented as-
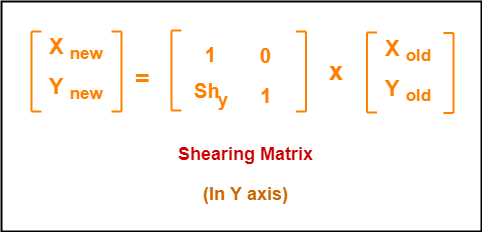
For homogeneous coordinates, the above shearing matrix may be represented as a 3 x 3 matrix as-
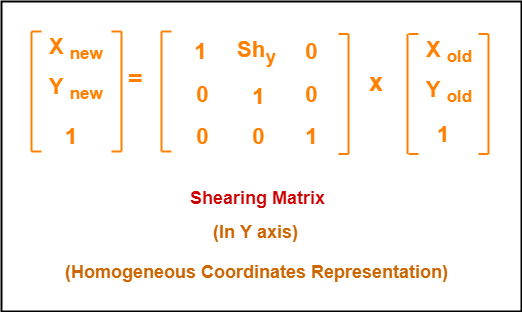
PRACTICE PROBLEMS BASED ON 2D SHEARING IN COMPUTER GRAPHICS-
Problem-01:
Given a triangle with points (1, 1), (0, 0) and (1, 0). Apply shear parameter 2 on X axis and 2 on Y axis and find out the new coordinates of the object.
Solution-
Given-
- Old corner coordinates of the triangle = A (1, 1), B(0, 0), C(1, 0)
- Shearing parameter towards X direction (Shx) = 2
- Shearing parameter towards Y direction (Shy) = 2
Shearing in X Axis-
For Coordinates A(1, 1)
Let the new coordinates of corner A after shearing = (Xnew, Ynew).
Applying the shearing equations, we have-
- Xnew = Xold + Shx x Yold = 1 + 2 x 1 = 3
- Ynew = Yold = 1
Thus, New coordinates of corner A after shearing = (3, 1).
For Coordinates B(0, 0)
Let the new coordinates of corner B after shearing = (Xnew, Ynew).
Applying the shearing equations, we have-
- Xnew = Xold + Shx x Yold = 0 + 2 x 0 = 0
- Ynew = Yold = 0
Thus, New coordinates of corner B after shearing = (0, 0).
For Coordinates C(1, 0)
Let the new coordinates of corner C after shearing = (Xnew, Ynew).
Applying the shearing equations, we have-
- Xnew = Xold + Shx x Yold = 1 + 2 x 0 = 1
- Ynew = Yold = 0
Thus, New coordinates of corner C after shearing = (1, 0).
Thus, New coordinates of the triangle after shearing in X axis = A (3, 1), B(0, 0), C(1, 0).
Shearing in Y Axis-
For Coordinates A(1, 1)
Let the new coordinates of corner A after shearing = (Xnew, Ynew).
Applying the shearing equations, we have-
- Xnew = Xold = 1
- Ynew = Yold + Shy x Xold = 1 + 2 x 1 = 3
Thus, New coordinates of corner A after shearing = (1, 3).
For Coordinates B(0, 0)
Let the new coordinates of corner B after shearing = (Xnew, Ynew).
Applying the shearing equations, we have-
- Xnew = Xold = 0
- Ynew = Yold + Shy x Xold = 0 + 2 x 0 = 0
Thus, New coordinates of corner B after shearing = (0, 0).
For Coordinates C(1, 0)
Let the new coordinates of corner C after shearing = (Xnew, Ynew).
Applying the shearing equations, we have-
- Xnew = Xold = 1
- Ynew = Yold + Shy x Xold = 0 + 2 x 1 = 2
Thus, New coordinates of corner C after shearing = (1, 2).
Thus, New coordinates of the triangle after shearing in Y axis = A (1, 3), B(0, 0), C(1, 2).
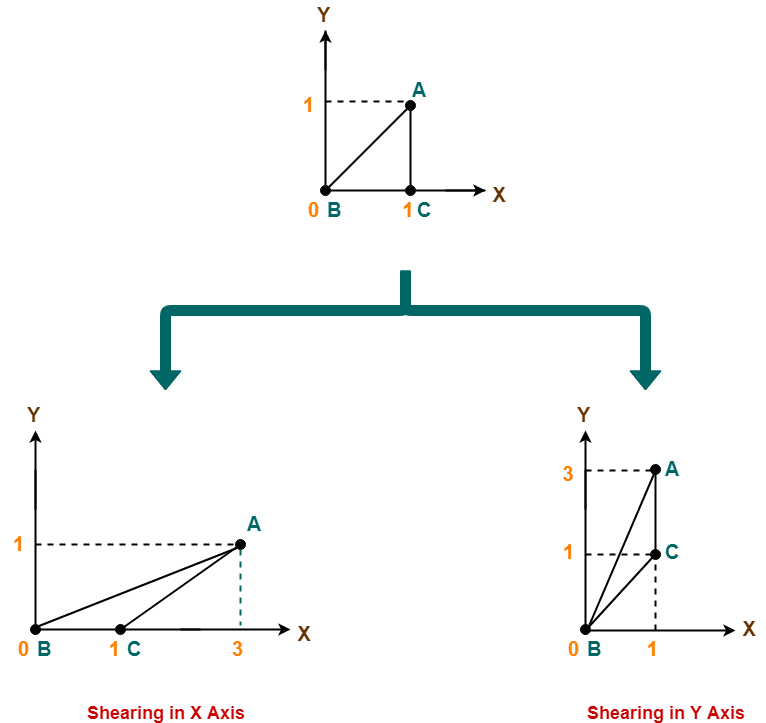
To gain better understanding about 2D Shearing in Computer Graphics,
Get more notes and other study material of Computer Graphics.
Watch video lectures by visiting our YouTube channel LearnVidFun.