Candidate Key and Primary Key

Tag: Candidate Key and Primary Key

Candidate Key | Finding Candidate Keys | Examples

Database Management System

Keys in DBMS-

Before you go through this article, make sure that you have gone through the previous article on Keys in DBMS.

We have discussed-

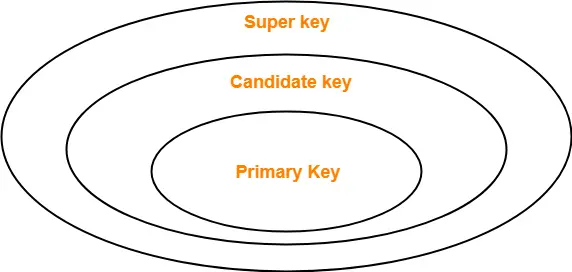
A key is a set of attributes that can identify each tuple uniquely in the given relation.
There are various types of keys in DBMS-

In this article, we will discuss how to find candidate keys of a given relation.

Candidate Key-

A candidate key may be defined as-

A set of minimal attribute(s) that can identify each tuple uniquely in the given relation is called as a candidate key.

A minimal super key is called as a candidate key.

For any given relation,

It is possible to have multiple candidate keys.
There exists no general formula to find the total number of candidate keys of a given relation.

Example-

Consider the following Student schema-

Student ( roll , name , sex , age , address , class , section )

Given below are the examples of candidate keys-

( class , section , roll )
( name , address )

These are candidate keys because each set consists of minimal attributes required to identify each student uniquely in the Student table.

Finding Candidate Keys-

We can determine the candidate keys of a given relation using the following steps-

Step-01:

Determine all essential attributes of the given relation.
Essential attributes are those attributes which are not present on RHS of any functional dependency.
Essential attributes are always a part of every candidate key.
This is because they can not be determined by other attributes.

Example

Let R(A, B, C, D, E, F) be a relation scheme with the following functional dependencies-

A → B

C → D

D → E

Here, the attributes which are not present on RHS of any functional dependency are A, C and F.

So, essential attributes are- A, C and F.

Step-02:

The remaining attributes of the relation are non-essential attributes.
This is because they can be determined by using essential attributes.

Now, following two cases are possible-

Case-01:

If all essential attributes together can determine all remaining non-essential attributes, then-

The combination of essential attributes is the candidate key.
It is the only possible candidate key.

Case-02:

If all essential attributes together can not determine all remaining non-essential attributes, then-

The set of essential attributes and some non-essential attributes will be the candidate key(s).
In this case, multiple candidate keys are possible.
To find the candidate keys, we check different combinations of essential and non-essential attributes.

We will further understand how to find candidate keys with the help of following problems.

The following practice problems are based on Case-01.

PRACTICE PROBLEMS BASED ON FINDING CANDIDATE KEYS-

Problem-01:

Let R = (A, B, C, D, E, F) be a relation scheme with the following dependencies-

C → F

E → A

EC → D

A → B

Which of the following is a key for R?

Also, determine the total number of candidate keys and super keys.

Solution-

We will find candidate keys of the given relation in the following steps-

Step-01:

Determine all essential attributes of the given relation.
Essential attributes of the relation are- C and E.
So, attributes C and E will definitely be a part of every candidate key.

Step-02:

Now,

We will check if the essential attributes together can determine all remaining non-essential attributes.
To check, we find the closure of CE.

So, we have-

{ CE }⁺

= { C , E }

= { C , E , F } ( Using C → F )

= { A , C , E , F } ( Using E → A )

= { A , C , D , E , F } ( Using EC → D )

= { A , B , C , D , E , F } ( Using A → B )

We conclude that CE can determine all the attributes of the given relation.

So, CE is the only possible candidate key of the relation.

Thus, Option (B) is correct.

Total Number of Candidate Keys-

Only one candidate key CE is possible.

Total Number of Super Keys-

There are total 6 attributes in the given relation of which-

There are 2 essential attributes- C and E.
Remaining 4 attributes are non-essential attributes.
Essential attributes will be definitely present in every key.
Non-essential attributes may or may not be taken in every super key.

So, number of super keys possible = 2 x 2 x 2 x 2 = 16.

Thus, total number of super keys possible = 16.

Problem-02:

Let R = (A, B, C, D, E) be a relation scheme with the following dependencies-

AB → C

C → D

B → E

Determine the total number of candidate keys and super keys.

Solution-

We will find candidate keys of the given relation in the following steps-

Step-01:

Determine all essential attributes of the given relation.
Essential attributes of the relation are- A and B.
So, attributes A and B will definitely be a part of every candidate key.

Step-02:

Now,

We will check if the essential attributes together can determine all remaining non-essential attributes.
To check, we find the closure of AB.

So, we have-

{ AB }⁺

= { A , B }

= { A , B , C } ( Using AB → C )

= { A , B , C , D } ( Using C → D )

= { A , B , C , D , E } ( Using B → E )

We conclude that AB can determine all the attributes of the given relation.

Thus, AB is the only possible candidate key of the relation.

Total Number of Candidate Keys-

Only one candidate key AB is possible.

Total Number of Super Keys-

There are total 5 attributes in the given relation of which-

There are 2 essential attributes- A and B.
Remaining 3 attributes are non-essential attributes.
Essential attributes will be definitely present in every key.
Non-essential attributes may or may not be taken in every super key.

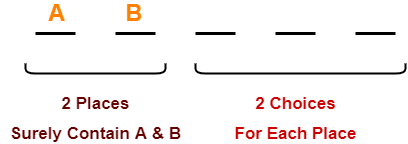
So, number of super keys possible = 2 x 2 x 2 = 8.

Thus, total number of super keys possible = 8.

Problem-03:

Consider the relation scheme R(E, F, G, H, I, J, K, L, M, N) and the set of functional dependencies-

{ E, F } → { G }

{ F } → { I , J }

{ E, H } → { K, L }

{ K } → { M }

{ L } → { N }

What is the key for R?

{ E, F }
{ E, F, H }
{ E, F, H, K, L }
{ E }

Also, determine the total number of candidate keys and super keys.

Solution-

We will find candidate keys of the given relation in the following steps-

Step-01:

Determine all essential attributes of the given relation.
Essential attributes of the relation are- E, F and H.
So, attributes E, F and H will definitely be a part of every candidate key.

Step-02:

Now,

We will check if the essential attributes together can determine all remaining non-essential attributes.
To check, we find the closure of EFH.

So, we have-

{ EFH }⁺

= { E , F , H }

= { E , F , G , H } ( Using EF → G )

= { E , F , G , H , I , J } ( Using F → IJ )

= { E , F , G , H , I , J , K , L } ( Using EH → KL )

= { E , F , G , H , I , J , K , L , M } ( Using K → M )

= { E , F , G , H , I , J , K , L , M , N } ( Using L → N )

We conclude that EFH can determine all the attributes of the given relation.

So, EFH is the only possible candidate key of the relation.

Thus, Option (B) is correct.

Total Number of Candidate Keys-

Only one candidate key EFH is possible.

Total Number of Super Keys-

There are total 10 attributes in the given relation of which-

There are 3 essential attributes- E, F and H.
Remaining 7 attributes are non-essential attributes.
Essential attributes will be definitely present in every key.
Non-essential attributes may or may not be taken in every super key.

So, number of super keys possible = 2 x 2 x 2 x 2 x 2 x 2 x 2 = 128.

Thus, total number of super keys possible = 128.

Problem-04:

Consider the relation scheme R(A, B, C, D, E, H) and the set of functional dependencies-

A → B

BC → D

E → C

D → A

What are the candidate keys of R?

AE, BE
AE, BE, DE
AEH, BEH, BCH
AEH, BEH, DEH

Solution-

We will find candidate keys of the given relation in the following steps-

Step-01:

Determine all essential attributes of the given relation.
Essential attributes of the relation are- E and H.
So, attributes E and H will definitely be a part of every candidate key.

The only possible option is (D).

Thus, Option (D) is correct.

Next Article- Functional Dependency in DBMS

Get more notes and other study material of Database Management System (DBMS).

Watch video lectures by visiting our YouTube channel LearnVidFun.

Types of Keys in DBMS | Definitions | Examples

Database Management System

Keys in DBMS-

A key is a set of attributes that can identify each tuple uniquely in the given relation.

Also read- Attributes in DBMS

Different Types Of Keys in DBMS-

There are following 10 important keys in DBMS-

Super key
Candidate key
Primary key
Alternate key
Foreign key
Partial key
Composite key
Unique key
Surrogate key
Secondary key

NOTE-

Before proceeding further, Kindly note-

The terms ‘relation’ and ‘table’ are used interchangeably.
The terms ‘tuple’ and ‘record’ are used interchangeably.

So, don’t get confused!

1. Super Key-

A super key is a set of attributes that can identify each tuple uniquely in the given relation.
A super key is not restricted to have any specific number of attributes.
Thus, a super key may consist of any number of attributes.

Example-

Consider the following Student schema-

Student ( roll , name , sex , age , address , class , section )

Given below are the examples of super keys since each set can uniquely identify each student in the Student table-

( roll , name , sex , age , address , class , section )
( class , section , roll )
(class , section , roll , sex )
( name , address )

NOTE-

All the attributes in a super key are definitely sufficient to identify each tuple uniquely in the given relation but all of them may not be necessary.

2. Candidate Key-

A minimal super key is called as a candidate key.

A set of minimal attribute(s) that can identify each tuple uniquely in the given relation is called as a candidate key.

Example-

Consider the following Student schema-

Student ( roll , name , sex , age , address , class , section )

Given below are the examples of candidate keys since each set consists of minimal attributes required to identify each student uniquely in the Student table-

( class , section , roll )
( name , address )

NOTES-

All the attributes in a candidate key are sufficient as well as necessary to identify each tuple uniquely.
Removing any attribute from the candidate key fails in identifying each tuple uniquely.
The value of candidate key must always be unique.
The value of candidate key can never be NULL.
It is possible to have multiple candidate keys in a relation.
Those attributes which appears in some candidate key are called as prime attributes.

3. Primary Key-

A primary key is a candidate key that the database designer selects while designing the database.

Candidate key that the database designer implements is called as a primary key.

NOTES-

The value of primary key can never be NULL.
The value of primary key must always be unique.
The values of primary key can never be changed i.e. no updation is possible.
The value of primary key must be assigned when inserting a record.
A relation is allowed to have only one primary key.

Remember-

4. Alternate Key-

Candidate keys that are left unimplemented or unused after implementing the primary key are called as alternate keys.

Unimplemented candidate keys are called as alternate keys.

5. Foreign Key-

An attribute ‘X’ is called as a foreign key to some other attribute ‘Y’ when its values are dependent on the values of attribute ‘Y’.
The attribute ‘X’ can assume only those values which are assumed by the attribute ‘Y’.
Here, the relation in which attribute ‘Y’ is present is called as the referenced relation.
The relation in which attribute ‘X’ is present is called as the referencing relation.
The attribute ‘Y’ might be present in the same table or in some other table.

Example-

Consider the following two schemas-

Here, t_dept can take only those values which are present in dept_no in Department table since only those departments actually exist.

NOTES-

Foreign key references the primary key of the table.
Foreign key can take only those values which are present in the primary key of the referenced relation.
Foreign key may have a name other than that of a primary key.
Foreign key can take the NULL value.
There is no restriction on a foreign key to be unique.
In fact, foreign key is not unique most of the time.
Referenced relation may also be called as the master table or primary table.
Referencing relation may also be called as the foreign table.

6. Partial Key-

Partial key is a key using which all the records of the table can not be identified uniquely.
However, a bunch of related tuples can be selected from the table using the partial key.

Example-

Consider the following schema-

Department ( Emp_no , Dependent_name , Relation )

Emp_no	Dependent_name	Relation
E1	Suman	Mother
E1	Ajay	Father
E2	Vijay	Father
E2	Ankush	Son

Here, using partial key Emp_no, we can not identify a tuple uniquely but we can select a bunch of tuples from the table.

7. Composite Key-

A primary key comprising of multiple attributes and not just a single attribute is called as a composite key.

8. Unique Key-

Unique key is a key with the following properties-

It is unique for all the records of the table.
Once assigned, its value can not be changed i.e. it is non-updatable.
It may have a NULL value.

Example-

The best example of unique key is Adhaar Card Numbers.

The Adhaar Card Number is unique for all the citizens (tuples) of India (table).
If it gets lost and another duplicate copy is issued, then the duplicate copy always has the same number as before.
Thus, it is non-updatable.
Few citizens may not have got their Adhaar cards, so for them its value is NULL.

9. Surrogate Key-

Surrogate key is a key with the following properties-

It is unique for all the records of the table.
It is updatable.
It can not be NULL i.e. it must have some value.

Example-

Mobile Number of students in a class where every student owns a mobile phone.

10. Secondary Key-

Secondary key is required for the indexing purpose for better and faster searching.

Next Article- Finding Candidate Keys of Given Relation

Get more notes and other study material of Database Management System (DBMS).

Watch video lectures by visiting our YouTube channel LearnVidFun.